
The general theory is illustrated and expanded using the examples of curves and surfaces. do Carmo 3.5 / 5 ( 2 ratings ) About this ebook One of the most widely used texts in its field, this volume introduces the differential geometry of curves and surfaces in both local and global aspects.
Differential geometry curves and surfaces full#
The authors begin with the necessary tools from analysis and topology, including Sard's theorem, de Rham cohomology, calculus on manifolds, and a degree theory. Differential Geometry of Curves and Surfaces: Revised and Updated Second Edition Show full title By Manfredo P. This book is an introduction to modern differential geometry. Cap, Monatshefte für Mathematik, Vol.Differential Geometry: Manifolds, Curves, and Surfacesīackground - Differential Equations - Differentiable Manifolds - Partitions of Unity, Densities and Curves - Critical Points - Differential Forms - Integration of Differential Forms - Degree Theory - Curves: The Local Theory - Plane Curves: The Global Theory - A Guide to the Local Theory of Surfaces in R3 - A Guide to the Global Theory of Surfaces - Bibliography - Index of Symbols and Notations - Index … A distinctive feature of the book is that, apart from a large number of exercises, it also contains many original problems with outlines of solutions.” (A. Only a basic background in analysis and linear algebra is needed to follow the presentation. “This book, written by one of the Russian masters, offers a comprehensive introduction to classical differential geometry of curves and surfaces. "Toponogov’s ‘concise guide’ to elementary differential geometry has the potential to be a useful reference and/or review book …. Numerous illustrations make the reading enjoyable." (Wolfgang Kühnel, Mathematical Reviews, Issue 2006 m) … the book is very welcome since it is an original contribution in various aspects and gives a number of geometric insights …. … the book is rich in geometry and concrete examples. Differential geometry of curves & surfaces / Main Author: Carmo, Manfredo Perdigo do (Author) Format: Book Language: English Edition: Revised & updated. … It can be recommended for first-year graduate students and also for use in the classroom. "This book by the late author covers … the subjects which are normally taught in a course on the differential geometry of curves and surfaces. Differential Geometry of Curves and Surfaces: A Concise Guide presents traditional material in this field along with important ideas of Riemannian geometry. A distinctive feature of the book is a large collection (80 to 90) ofnonstandard andoriginalproblems that introduce the student into the real world of geometry. In the last case, the formulations are discussed in detail. The focus is not on mathematical rigor but rather on collecting some bits and pieces of the very pow- erful machinery of manifolds and post-Newtonian calculus'. Bernstein’s theorem about saddle surfaces. These notes are an attempt to summarize some of the key mathe- matical aspects of dierential geometry, as they apply in particular to the geometry of surfaces in R3. Pogorelov’s theorem about rigidity of convex s- faces, and S.N. Aleksandrov’s comparison theorem about the angles of a triangle on a convex 1 surface, formulations of A.V. The second stream contains more dif?cult and additional material and for- lations of some complicated but important theorems, for example, a proof of A.D. It includes the whole of Chapter 1 except for the pr- lems (Sections 1.5, 1.7, 1.10) and Section 1.11, about the phase length of a curve, and the whole of Chapter 2 except for Section 2.6, about classes of surfaces, T- orems 2.8.1–2.8.4, the problems (Sections 2.7.4, 2.8.3) and the appendix (S- tion 2.9).

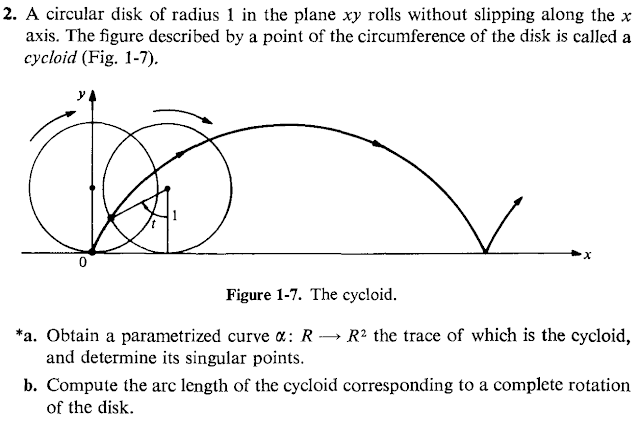
It contains a small number of exercises and simple problems of a local nature.
The ?rst stream contains the standard theoretical material on differential ge- etry of curves and surfaces. The material is given in two parallel streams. This concise guide to the differential geometry of curves and surfaces can be recommended to ?rst-year graduate students, strong senior students, and students specializing in geometry.


 0 kommentar(er)
0 kommentar(er)
